Ever since the covid19 stay-at-home orders were relaxed for my state, I have been struggling to find some rules to guide me as we try to safely host small gatherings with qualified friends (today’s rules: outdoors, safe-distancing, maximum of two guests–who have also been in semi-quarantine).

I’d like to know “what is my risk?” after encountering N people in a day and spending a certain amount of time with each.  In particular, if I interact with store clerks for a few minutes each, walk or bicycle past maybe a hundred people, or sit in a (sparse) movie theater with a few dozen others for two hours, what risks am I taking?  I want to put it in relative terms with the risk I willingly accept when I drive a few miles for an everyday errand.

There seems to be growing evidence that the covid virus is transmitted primarily through the air, and less frequently by fomites, virus particles lingering on surfaces.  The airborne channels include “droplets”, which float for a while but eventually drop to the floor, and “aerosols”, which stay aloft among the air molecules.  We encounter both of these when confined in a room with an infectious person.

I consider the situation to be analogous to someone wearing perfume, or smoking:  if you can smell the perfume or smoke, you are inhaling particles, diffused across the air from that person to your nose.  I also will use the concept of a critical dose– the amount of virus needed to contract the disease.  One must breathe in enough odorless “covid scent particles” to become infected, which is a time*intensity dimension, something familiar in photography where both the exposure time and the intensity of the light factor in to making a mark on the film or sensor.  The exposure time is easy enough to quantify, but the covid perfume “intensity” needs to be established.

I propose several intensity levels.  The lowest is just breathing the air in a room shared with another person breathing the same air.  Next is sharing the airspace while talking (no mask).  Above that is engaging in stronger aerosol-generating activities: singing and shouting.  The highest level might be coughing and sneezing in the same interior space.  I am unfamiliar with the research in this field, so I will invent my own scale for their relative values and update them as I learn more:

Breathing: 1
Talking: 2
Singing: 4
Sneezing: 8

The “compartmental model” in epidemiology has the concept of the rate of new infections, beta, the “average number of contacts per person per time, multiplied by the probability of disease transmission in a contact between a susceptible and an infectious subject”

Recasting this, I interpret it to mean that beta is the ratio of exposure, e, in person-intensity-hours to the “critical infectious exposure”, e_c, the amount of person-intensity-hours that will trigger an infection.

As an example, let’s say you are in a room with a random person.  The probability that this person is infected (or that YOU are infected for that matter) is I/N, the number of infected people in your region to its population.  Your exposure is the number of hours you spend in the same room, times the interaction intensity, one if silent, two if conversing etc.  Your risk of infection is the ratio of your exposure to the critical exposure, times the likelihood that the other person is infected.

e = sum (t_n * i_n)

P(infection) = (e/e_c) * (I/N)

Where n indexes the number of people, t is the duration time in contact with them, i is the intensity level.  The total is e, the exposure in person-intensity-hour units

It is not too hard to estimate our exposure with the outside world (number of people we encounter, how long, what our interaction intensity is), but how do we know what the critical infection exposure is?

We have some clues.  There are cases of covid transmissions from birthdays, funerals and dinner parties.  There is the famous case of the choir practice.  These suggest that the critical exposure is in the neighborhood of a few hours at an intensity level of talking or singing.

I approach this inspired by the social contact exposure data from a paper I found: Mixing patterns between age groups in social networks.  From this paper, we learn that the average number of people we interact with in a day is about 16 (on average; for adults age 20 – 60, the number is a little above 20).  

These statistics should factor into the rate that the disease spreads, in particular the parameter R₀, the number of people subsequently infected by one infectious person.  Say there is a single infected person in the population.  They are infectious for some period of time and interact with some number of people.

Although we quarantine for 14 days to be safe, I suspect that the effective infectious period is less than that, certainly if we are instructed to isolate when we have symptoms, so say the infectious period is seven days.  If we take the face value that an infected subject interacts with 16 people per day, then 7*16=112 will be further infected.  This is an R₀ value of 112, which is beyond even the most virulent transmission of measles.  Obviously, this is not the way to estimate R₀.

For many of us, most of our daily interactions are with the same individuals.  Only the shortest interactions are unique, the others are with our classmates, coworkers and family. If the number of contacts we make are the same each day, then the infection rate depends only on our one-day contacts, and R₀ becomes 16, the average number of daily contacts.  This still exceeds the observed value.  I submit that it is because the exposure distribution matters.

This figure shows the relationship between number and duration of contacts within a (simulated) population.  It includes a histogram of contact durations, and the cumulative number of contacts during a typical day (based on data from figure 5 in the cited paper).  

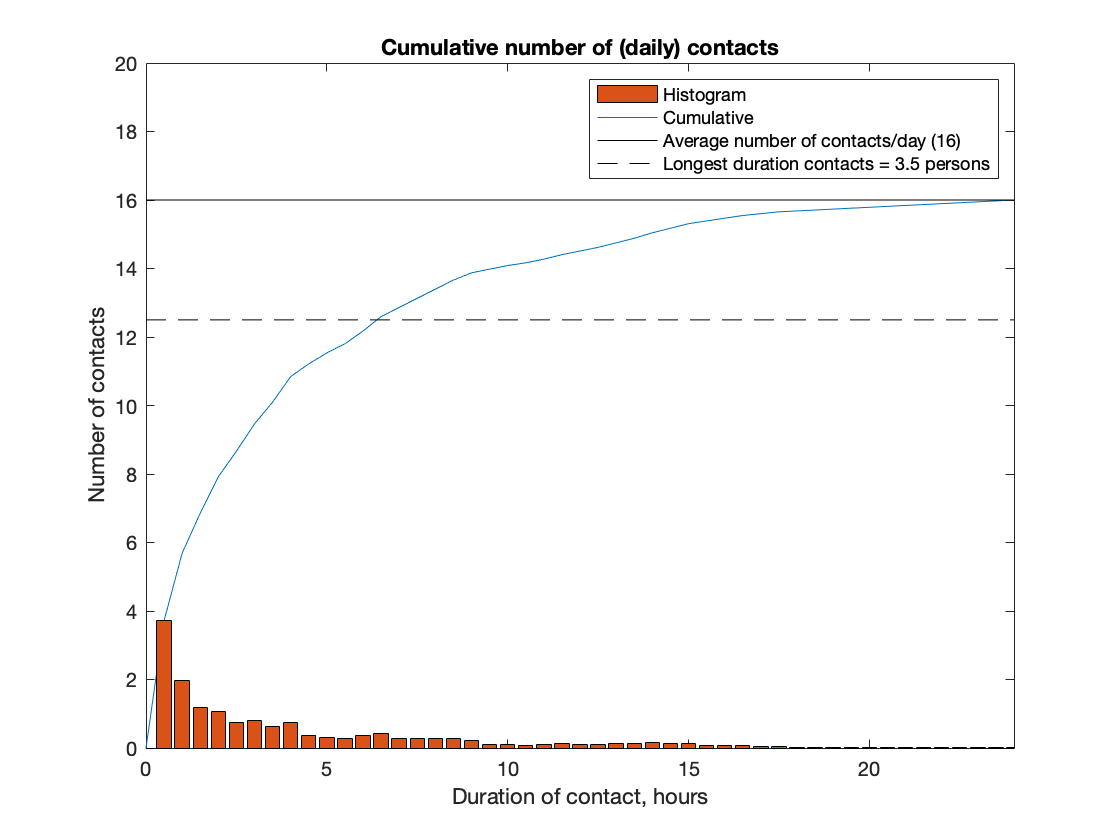
The histogram has been normalized such that its cumulative total reaches the average number of contacts we have each day.  Most of our daily contacts are of short duration.  Four contacts per day are for less than 30 minutes; six are for less than an hour. 

In the early days of covid, before there were widespread social distancing rules, the “natural” transmission rate had a value of R₀ between 3 and 4.  We can use this to estimate what the critical exposure level is.  What exposure results in 3.5 new infections? 

If the critical exposure was 1 minute, then all longer interactions would result in an infection; R₀ would be 16, as before: all the people that the infected person met over the day (which we are now assuming are the same 16 people encountered each day during the infectious period).  If the critical exposure was over one hour, the 6 short duration contacts of an hour or less would not get the necessary exposure to become infected, and only 10 remaining people would be infected; R₀ drops to 10.  The exposure that results in the observed natural R₀, 3.5, can be found by looking where the cumulative number of contacts results in 3.5 people becoming infected (the level 16 – 3.5 = 12.5).  This occurs at a duration time of six hours and longer, a period that represents usual activity times in classrooms or offices, and certainly the time at home with family members. 

This analysis suggests that we should not be concerned with our few short duration contacts; they just don’t add up to enough exposure to trigger an infection.  On the other hand, we have not yet taken into account the exposure intensity.  If a short duration contact was at a high enough intensity level, it could reach the critical exposure (dose) to become infected.

How do we include the concept of exposure intensity?  I propose multiplying the duration histogram frequencies by the intensity of the contact for each duration bin.  The rationale for this is that a duration bin that includes n contacts at intensity level 2 is equivalent to 2n contacts at intensity level 1.  They represent the same exposure.  Essentially this normalizes the number of contacts in the duration bin to a common intensity level, one.  Once we have the bins holding exposure values, we can perform the same normalization of the cumulative histogram as before to yield a sum representing the 16 people on average, that we encounter each day.

Unfortunately we do not have the intensity values for the contacts, so I will make some assumptions.  We expect that the short duration contacts are probably interactions that involve talking, intensity level 2.  And the long duration contacts are probably more passive (sleeping, watching TV, reading in the same room etc), intensity level 1.  There may be some intermediate contact durations that are more intense (cheering at sporting events, choir practice, etc), but with the lack of data and in the interests of keeping a conservative estimate, I do not use this level.  Instead, I split the duration bins into two groups; the shorter durations are scaled by two, representing talking, the longer ones remain weighted by one, and represent breathing in the same airspace.  

The split is initially set at 12 hours, which happens to match the average amount of time spent at home (certainly 8 hours of sleeping does not involve much talking).  When this weighting is applied, the duration at which 3.5 infections result drops to five person-intensity-hours instead of six.  If the split between short high intensity, and long low intensity durations is taken to be 6 hours instead of 12, the exposure that yields an R0 of 3.5 drops to 4 person-intensity-hours.

This means that the critical exposure is, conservatively, four person-intensity-hours.  We can now use this as a “budget” and estimate how much time and at what intensity level we are willing to spend in various activities.  If we want to sing in a quartet (intensity level 4), we can do so, but only for a duration of 20 minutes to not exceed the critical exposure (3 * 4 * 20/60 = 4 person-intensity-hours).  We can also estimate how long a movie we can watch in a theater with an audience of 40; unfortunately, it’s only 6 minutes (40 * 1 * 6/60).

These are the risks if the people we are engaging with are all infected.  Obviously not all of them are.  The probability of contracting the virus depends on the relative exposure, times the likelihood that a person in the population is infected.  If you want to assume that everyone is infected, then the critical exposure budget is the way to live your life.

This is too restrictive for me, and not an accurate representation of the true risk.  When we multiply by the likelihood of a person in the neighborhood being infected, the risks come down substantially.  In my county, one of the worst-hit in Minnesota, the current active confirmed infections are about 2000 (18 July 2020).  The total number of infections (including untested and unconfirmed) is some multiple of this; the estimate by IHME is about 2X, but not all of them are out in the population, maybe half of them are because they are pre-symptomatic, asymptomatic, undiagnosed, or diagnosed but not isolating.  So half of 4000 current infections are out there, somewhere, in a population of 1.25 million, which means that roughly one out of 600 people in the county is infected.  

Another way to estimate this is to obtain the latest number of daily new confirmed cases, multiply by the hidden infections factor to obtain an estimate for the total number of new cases each day.  Since the new cases have been “at large”, and infectious sometimes without symptoms, multiply by the number of days that the newly identified cases have been out and about prior to being isolated (including those that never get officially diagnosed).  Finally, divide by the population.  The recent daily confirmed cases in Hennepin County are 200.  Twice that is 400, and seven days worth is 2800.  The population is 1.25 million, so this is roughly one in 450 people.

The two estimates are consistent, given the uncertainties in making them, so in the interest of being cautious, I will use 1 in 250.  This is not zero, so I can’t ignore the risk, but it is also relatively unlikely that I will encounter one of them in my limited outings.  This means that my probability of infection is not my exposure ratio, but rather 250 times that, and my exposure budget of 4 becomes 1000 person-intensity-hours.  I can spend two hours in a conference hall with 500 people (two of whom are likely to be infected) before reaching the critical exposure.  I can spend four hours on a plane with 250 passengers (one of whom will likely be infected).  

The flaw in these examples is that I am not splitting the exposure time equally with all of the other people in the room.  Instead, there will be one or two individuals sitting next to me for the full duration.  If either or both happen to be the one (or two) who are infectious, my exposure will exceed the critical exposure.  My exposure budget of 1000 needs to be spread equally among the people I am with, so that no one individual encounter exceeds the critical exposure.  

This is something that can be done when the gathering of people is fluid, such as at a trade show or farmer’s market with many vendors.  It also suggests that there may be a way to “dilute” the effects of crowds at sporting events, by say, not assigning seats, but requiring everyone to “keep moving”.  If no one spends more than the critical exposure time next to any one other person, nobody gets a full infectious dose of virus.  This may also explain the lack of an infection spike after the George Floyd protests.  In addition to being outdoors, it is possible that the protesters mingled and mixed enough to keep the contact durations shorter than the critical exposure.

The risks of covid infection can be compared to the risks of driving.  If one is involved in a traffic accident crash, there is a range of consequences from no injury, to serious injury, to death.  Fourteen percent of traffic crashes result in minor or serious injuries, likely involving a trip to the hospital emergency room.  The fraction of covid cases that require hospitalization is about 10%, higher if you are older.  These are roughly the same.

Of those hospitalized with covid however, a third will die.  Out of the car crashes, the fraction (of injuries) that result in death is a tenth of that, 3.4%.

The risk of having a crash is proportional to the number of miles you drive, roughly one crash every one million miles.  The risk that you obtain a confirmed covid infection in my county has recently been reported at 150 per million people, per day.  So the current risk of contracting covid in my county is the same risk as having a crash while driving 150 miles (Minneapolis to Duluth).  I have made this trip many times, but not daily. It would take ten years before reaching a 50% chance of having a crash.  

To summarize:

If the primary mechanism for infection is inhaling aerosols and droplets, we can focus on the time we spend sharing the air with others and the intensity of how we generate droplets and aerosols.

Intensity of droplet and aerosol creation can be assigned to different activities.  A scale was proposed, but no quantitative data has been obtained yet to calibrate it.

A critical exposure of time and intensity is needed to obtain an infectious dose of virus.

The natural transmission rate R0 of covid, based on estimates prior to social distancing rules, is around 3.5.

An estimate obtained by an interaction model of a population prior to social distancing suggests that the critical exposure with an infectious person is about four person-intensity-hours (four hours of passive activity in the same room).

The odds that a random person is infectious can be estimated from the daily new case count.  I multiply by 28 and divide by the population. 

Combining these results allows an estimate for risk of infection when participating in some activity involving others.  Count the number of people, the duration, and the intensity level.  Divide by four (the critical exposure).  Multiply by the odds of a person being currently infectious.

If the calculated risk of infection for the activity is expressed as n chances per million, n is the number of miles one would drive for the same risk of being in a car crash. 

Going through this exercise has helped me gain some perspective on the risks I take when interacting with others.  I no longer worry about the sidewalk encounters while walking or bicycling, the exposure is just too small.  And interactions with store clerks is also not worrisome—except for the poor clerk, whose cumulative exposure could become significant.

Other situations also yield low risks, such as dining with my partner on a patio with a dozen other tables at generous spacings.  Unfortunately, at some point in my risk assessments, even though I know better and would readily undertake the equivalent driving trip, the contemplation of the risk of covid infection is emotionally much higher.